Pistol Thumb Position

In general, when shooting a semi-automatic pistol there are four possible positions for the shooting hand thumb.

Number 1:  The thumb wraps around the grip and touches the index finger making a fist.  This thumb position increases the ability to apply the strongest grip force.  If the weak hand is used, its thumb clamps over the shooting hand thumb, increasing retention.  Police are taught this most secure grip to support weapon retention during a scuffle.

Number 2:  The thumb extends straight forward, parallel to the barrel.  This is currently the popular mode with the practical pistol and target shooting folks.  If the weak hand is used, i.e. not bulls-eye, its thumb is placed parallel to and just below the strong hand thumb.  Grip force is applied with the fingers alone.

   Although I normally shoot position one, I recently discovered an important use for the second.  Very light micro 380 compacts like the Ruger LCP, Keltec P3AT, and S&W Bodyguard 380 allow only two fingers on the grip and as a result squirm significantly in your hand from recoil when shot.  The problem is that with position number one and the small grip your thumb is directly on the magazine release and, on pistols like the S&W with a tall release button, can drop the magazine when the pistol is shot.  Position two solves this problem.  BTW I have never had this problem on a full sized pistol.

Number 3:  Years ago when center fire bulls-eye was shot almost exclusively with 1911s, competitors often placed their shooting hand thumb on top of the manual safety.  Some even had custom safeties made with shields extending up along the side of the slide to protect their thumbs from the slide when shooting.  This appears to have fallen out of favor over the years.

Number 4:  The Flag.  In this position the shooter points their thumb straight up.  I don’t get it but there are older shooters who shoot this way and are better than I am so who am I to comment?

The spambots are getting out of hand : Please leave comments, friend, using the post in my comments category. 🙂

Laundry Sanitizer

You wash your towels and washcloths in hot water and detergent, and then tumble dry on high heat.  They come out smelling perfectly fresh and stay that way until the first time they get wet.  Then you notice a musty odor on what were “clean” towels.  You are the victim of microorganisms that go dormant when dry, activate when damp, and are untouched by detergents.  In the past true laundry sanitizers were absent from consumer markets.  Consumers were limited to products that removed sweat odors from gym clothes. Effective sanitizers are now readily available at the supermarket.

The best we’ve found is “Lysol Laundry Sanitizer Free & Clear”.  This is a clear liquid that you add to the washer just like fabric softener.  It has no strong odor, unlike some older Lysol disinfectants and will not leave any residue on your wash.  We use it on every washer load.  After a few wash cycles you will notice that your damp towels are much fresher than before.

The spambots are getting out of hand : Please leave comments, friend, using the post in my comments category. 🙂

M1911 Extractors

The Colt 1911 is a great pistol.  It has superb ergonomics, excellent accuracy, a svelte body, a long history of supremacy as a fighting pistol, and a magnificent trigger.  Many people find that they are simply more accurate with a 1911 than with other more common designs.

            The main reliability weakness of the 1911 centers around the internal extractor.  I have six 1911’s and generally any problems are due to extractor adjustment.  The extractor tension is critical.  A low extractor tension results in failures to extract and stovepipes, while tension that is too high results in failures to feed.  Every gunsmith has their own method to adjust this tension using various rules of thumb.  Even 1911 fanatics will admit that the extractor needs to be adjusted every 500 rounds or so while pistols with external extractors and separate springs will go thousands of rounds with no extractor problems.

            Various manufacturers of 1911 clones have chosen to switch to an external extractor.  The first was Smith & Wesson with the SW1911.  SIG came out with a 1911 with an external extractor.  Now even Staccato and Wilson Combat have external extractors on some models.

            A puzzling fact is that in the 1980’s Army testing comparing the 1911 to the Beretta and SIG, the 1911 had a fault in less than 200 rounds while the Beretta and SIG ran thousands of rounds without a problem.  But, in 1911, the original John Browning design ran 6000 rounds without a problem, far better than competing pistols like the Savage and Lugar.  A possible explanation is contained in this product description from the Cylinder&Slide web site:

 “Our Ultimate 45 ACP 1911 Extractor is the only extractor that is made from the correct spring steel called out by the military print. I have had every extractor that I could purchase tested to determine the steel alloy. All of the extractors that I had tested were made from the same incorrect steel. The Ultimate extractor has the correct nose shape and the proper radii for the most reliable function. The Ultimate  extractor will maintain its tension long after the others have failed. Tensioning instructions are included with every Ultimate Extractor. Every Ultimate Extractor is Rockwell tested to insure correct heat treatment. The Ultimate Extractor is not only heat treated it is Austempered for maximum wear resistance.”

Here is a more detailed description of this issue from Cylinder & Slide and what they had to go through to make these:

https://cylinderslide.com/PDFarticles/ExtractorHistory.pdf

By the way, if you need to replace the thumb safety on a Smith & Wesson SW1911, a “standard” safety will not fit as it interferes with the sear.  Smith apparently changed a critical dimension in the frame that affected this.  Their manufacturing solution was to buy commercial safeties and hand fit them to each pistol. The safety to sear interface in the 1911 is very critical and a replacement safety needs to be fitted by a gunsmith who thoroughly understands the geometry and interaction.  I don’t know if S&W has corrected this on their SW1911E models.

The spambots are getting out of hand : Please leave comments, friend, using the post in my comments category. 🙂

Relay Dynamics Puzzle

While measuring relay response times I noticed an unusual effect. I was working on a (relatively) high power pulse project using automotive relays. I was trying to maximize the repetition rate while maintaining adequate dwell time to charge the high voltage circuit. Measuring a typical 40 amp automotive relay with a reverse diode across the coil yielded the following delay times.

With diode: turn on: N.O. make – 5.0ms, turn off: N.O. break – 6.7ms.

The diode clamps the inductive spike from the coil by recirculating the current. The problem is with almost no voltage across the coil the current and thus the magnetic field takes a long time to decay. Hence the long break time. A standard remedy is to place a zener diode in series with the catch diode. Using a 12 volt zener with a 12 volt relay yields the following delay times.

With diode and zener: turn on: N.O. make- 5.6ms, turn off: N.O. break – 2.6ms.

A 61% reduction in turn off time. Great. But somehow, the turn on time which should have nothing to do with the diode/zener increased by 12%. Weird. After rechecking everything and some thought and other measurements I figured out what was going on.

When voltage is applied to the relay coil the inductance of the coil slows the build up of current and the resulting magnetic field. Once the field strength of the core exceeds the strength of the return spring, the armature starts moving. This takes 3.1ms with the diode and 3.7ms with the zener in repetitive mode. Then the armature takes 1.9ms to move to the N.O. contact. When coil voltage is removed the coil inductance tries to keep the current flowing by reversing the voltage.

For the diode version this reverse voltage is clamped t 0.6 volts which lets the coil current slowly decrease for 6.7ms when the field is low enough to release the armature which returns to rest under spring tension in another 1.5ms.

For the zener+diode version the reverse voltage is clamped at 12.6 volts which absorbs the coil energy much much more quickly. The coil inductance holds this voltage for 3.2ms and then decays for a few ms. The current has been decaying continuously and allows the N.O. contact to open after 2.6ms.

The turn on anomaly is due to the parasitic coil capacitance and the relay core hysteresis. With the simple diode circuit the coil is never exposed to reverse polarity current so the magnetic core simply tracks back and forth on the same side of its hysteresis curve. With the zener circuit, after the coil current decays to zero, the 12.6 volt reverse polarity across the coil has charged up the parasitic capacitance of the coil. This now discharges in a reverse current through the coil. This can be observed as the slow decay of the coil voltage from the 12.6 volt peak. This reverse polarity current moves the the iron core of the relay to the other side of its hysteresis curve. The next time the relay coil is energized the coil current has to ramp up enough to demagnetize the reversed core and remagnetize it in the forward direction before the armature can start to move. This causes the additional 0.6ms turn on delay.

The spambots are getting out of hand : Please leave comments, friend, using the post in my comments category. 🙂

Levi Jeans

For over 150 years Levi has been making work pants and after the initial sail cloth trousers for the 49ers, created the denim blue jeans that became the standard for construction workers, farmers, ranchers, hunters, and anyone who wanted heavy duty pants. For those 150 years Levi jeans always had seven belt loops, two in front, two on the sides, one in the middle of the back, and two flanking the middle loop half way to the sides. For over a century these seven loops have provided rugged support for Levi pants.

Apparently, like many long time companies that have forgotten their roots, Levi decided that their jeans are “Fashion Statements” rather than work pants. So some wonk the the accounting department decided to save a few cents by eliminating two belt loops in the back because none of the fashionistas needed them. Don’t worry about the gaps at the back, just go with the flow. Check out Levi’s web site to see what they are making now. While they may do well on Rodeo Drive, somehow I don’t see them being popular with the actual rodeo folks.

The spambots are getting out of hand : Please leave comments, friend, using the post in my comments category. 🙂

Softened Water Kills Plants

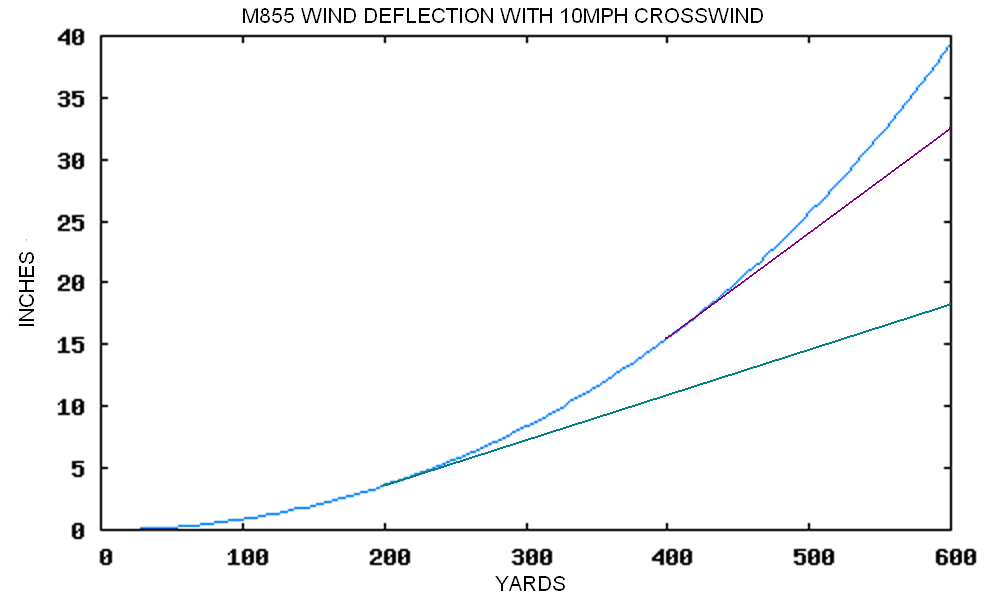
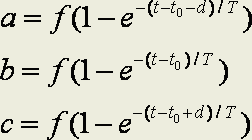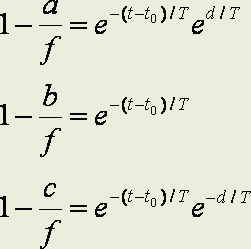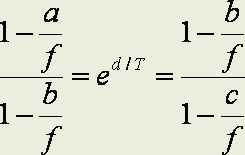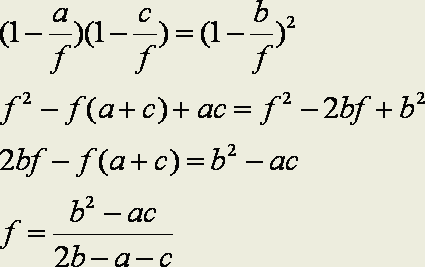Water softeners replace slightly soluble calcium and magnesium carbonate and sulfate salts in hard water, picked up from rocks like limestone and gypsum, with more soluble sodium salts. Since the sodium salts rinse away better, they do not leave significant water spots, lime, and scale and are harmless to people and animals, although some people can taste the sodium salts more than the hard water salts. They also improve the efficiency of soap. The used up sodium has to be replaced in the water softener by periodically adding salt pellets.

Plants, with the exception of salt water grasses, sea weed, and algae, did not evolve in an environment with sodium salts. If they are exposed to sodium salts the sodium blocks the pores used by plants to take up water and the plants die of thirst. This has been known for a long time. When the Romans finally destroyed the Carthaginians, they salted the ground in Carthage so nothing would ever grow there. The herbicide Roundup kills all plants but does not poison the ground and allows replanting three days later. Roundup 365 is intended for killing plants where no plants are ever desired as between driveway pavers. It is made by adding salts to regular Roundup.

All water softeners have bypass valves to switch back to hard water temporarily. The instructions tell you to switch to bypass when watering the lawn but never explain why. It seems this is to avoid wasting replacement salt pellets but it is actually to keep the softened water from killing the grass (and trees). I don’t know if it was a marketing department or legal staff that decided to not mention this product downside but this omission has spread to the entire industry.

I managed to kill a newly planted tree to learn this. The more I watered it, the drier it got. When you switch to hard water, there is still softened water in the house pipes. You will need to run that out before you turn on your hoses. You can do this by running a faucet near the part of the house where to hoses are for a couple of minutes. In my house the bathrooms are beyond the hoses with respect to the water softener so I can just flush a couple of toilets and wait for them to refill. Always switch to hard water bypass when using your hoses, even for cleaning, to keep softened water out of them.

The spambots are getting out of hand :  Please leave comments, friend, using the post in my comments category.  🙂

Beretta PX4 Upgrades

The Beretta PX4 is a high quality DA/SA hammer fired handgun. It is extremely reliable and with low recoil flip due to its rotating barrel lockup rather than the typical tilting barrel. Good as it is, Langdon Tactical offers a number of upgrades ranging from parts to complete pistols with custom triggers, safeties, and other goodies. Many of these upgrades are available as drop-in replacement parts.

I bought three of the minor upgrades, an 11# PX4 Chrome Silicon Hammer Spring, the Optimized Performance Trigger Bar, and the Stealth Lever Kit.

Langdon sells a range of hammer spring weights. 11# is the second lightest, which they recommend for all ammunition. Their 10# unit is recommended for American factory ammunition only as some European ammo has harder primers.

The effect of the 11# spring was to lower the double action pull from 10 pounds to 8-1/2 pounds. The single action trigger pull went from 5-1/2 pounds to 5 pounds. Both were noticeable differences. The new spring has functioned flawlessly.

As a Langdon video shows, on new guns it helps to “burnish” the hammer/sear surfaces by dry firing the pistol a few times while pressing on the back of the hammer. On mine this dropped the double action pull from 10-1/2 to 10 pounds all by itself.

The new trigger bar improves the trigger reset from about 0.24 inches to 0.07 inches. This helps a lot with split times.

The PX4 is a fairly large gun which helps with recoil and target reacquisition but makes it somewhat bulky. The two ambidextrous safety ears on the factory gun stick out making the gun even bulkier. Langdon’s stealth decockers are essentially flush to the slide and much easier to operate. They convert the standard PX4 to decocker only. As a carry option this eliminates the need to switch off a manual safety. Beretta offers a similar version of the PX4 but their levers still stick out.

Earnest Langdon makes these installations look easy in the videos on the Langdon Tactical web site but if you decide to do this you will want to watch their “Trigger Job in a Bag” video several times. For both you will want to study exactly how the trigger bar fits into the hammer/sear assembly in your gun before you start. There are also videos on the Langdon site and YouTube for the stealth decocker installation. Alternately just take it all to a gunsmith.

I bought the adjustable target sights from Beretta. These DEFINITELY require a gunsmith as the factory dovetail fixed sights are way too tight for ordinary tools.

The spambots are getting out of hand :  Please leave comments, friend, using the post in my comments category.  🙂

S&W 41 MAGAZINE LOADER

The “Mag Pal Speed Beez S&W Model 41 Clip Loader” from Amazon is a major aid to anyone with a Smith & Wesson Model 41 target pistol. Interestingly enough, this loader also works on Norinco TT Olympia target pistol magazines. Hope this helps someone out there.

It also works on the S&W 22A and the Ruger MK I-III. It’s looser than a dedicated Ruger loader which will not work with other 22’s in general.

The spambots are getting out of hand :  Please leave comments, friend, using the post in my comments category.  🙂

Sock Sorters

40 or 50 years ago there was a firm in Hong Kong manufacturing a small useful laundry accessory called a Sock Sorter. This was a one piece disk shaped clip designed to keep pairs of socks together during the laundry cycle.

The original, well designed product is on the left in brown. It is easy to use, with soft fingers that are easy on the socks. The original firm has long since gone by the wayside but other Chinese companies have picked up on this and are producing copies of a sort. Searching for “Sock Sorters” on Amazon brings up several. Unfortunately the copiers had apparently only seen pictures of the original and did not have physical access to samples. The copies appear to be made of polyethylene like the originals but have much stiffer fingers. The version in the center is more difficult to use and in the case of the item on the right in dark orange the result is too stiff and small to use with any but the thinnest of socks. In an effort to help any other budding entrepreneurs, herewith are the actual dimensions in inches:

Original:

Outside diameter 1.155

Finger Thickness 0.024

Ring Thickness 0.075

Inside diameter 0.922

Tip gap 0.179

————————–

Center (light orange):

Outside diameter 1.211

Finger Thickness 0.044

Ring Thickness 0.121

Inside diameter 0.970

Tip gap 0.181

————————-

Right (dark orange):

Outside diameter 1.103

Finger Thickness 0.042

Ring Thickness 0.121

Inside diameter 0.842

Tip gap 0.137

————————-

As an added benefit, the original at 0.466 grams uses 41% less material than the the 0.787 gram copy.

The spambots are getting out of hand :  Please leave comments, friend, using the post in my comments category.  🙂

Electronic Earmuff Noise

One ongoing source of annoyance is the omission of critical specifications by manufacturers. A prime example is electronic earmuff noise. This is the hissing sound that can be heard in most audio systems when you turn up the volume without other input and is dependent upon the design of the preamplifier circuit. Some common muffs have so much noise that the claim of being used to heighten hearing to detect quiet sounds is essentially meaningless. These also have a high enough noise level to be really annoying at volume levels suitable for range conversation. As a result you turn them off and gain nothing over cheaper passive muffs. This noise level could be specified in dB for a given condition just like the NRR rating or the maximum amplified level. Since it is not, it is impossible to tell before buying a pair whether or not the noise level is too high for usability. Unfortunately, even the low noise units do not specify this and are probably losing out on some sales as a result.

One example of the latter are the Honeywell Howard Leight Impact Sport (20dB NRR) and Impact Pro (30dB NRR) muffs. These are high quality muffs and are very quiet. At least the Pro says it can amplify animal sounds up to 5X. Both of these have similar low noise levels compared to some other brands. Once I discovered this using a friend’s pair, I bought two Impact Pro’s (one for the wife) and donated my existing electronic muffs.

My recommendation, if you are looking into electronic muffs, is either buy Howard Leight Impact Pro units or go to a sporting goods or firearms store in person where you can try out various brands.

The spambots are getting out of hand :  Please leave comments using the post in my comments category.  🙂